Thinking of XIX-century Great Britain many things come to mind such as romance novels with tormented love stories and ladies trussed in corsets and Victorian gowns. What is less familiar to our common imaginary is to localise such women in less romantic contexts like mathematics and science, let alone in something pioneering like computing.
Yet, the most unlikely stories make it to history, even more than those filled with passion “Pride and Prejudice”-style. Our main character is a lady, yes, who, however, liked numbers above anything else. She is Countess Ada Lovelace, the only legitimate child of Lord Byron and Annabella Milbanke, who conquered, hands down, the title of first-ever computer programmer.

Ada’s background is to be considered wealthy indeed, however, the life of an English woman in the 1800s was anything but easy. Ada never knew her father, her parents separated only after two months after she was born and her mother tried very hard to keep her from the “excessively poetic” (= irrational) traits of the Byron dynasty. Lady Byron was determined to give her daughter a different kind of life and she hired tutors and teachers who would teach Ada maths, music and French.
But the extraordinary opportunities given to Ada are not limited to studies alone: her wit and intelligence take her places in the London social panorama. During one iconic event in 1833 Ada meets Charles Babbage, renewed mathematician, and her life takes an unusual turn. Everybody is talking about Babbage’s “Difference Machine”, a tower of numbered wheels which could make calculations simply by turning a handle. Ada decides to go see Babbage’s creation for a demonstration and she’s incredibly fascinated by it, so much that the two scientists begin a correspondence in which they share thoughts about the potential of such machine and they discuss ways to improve it.


Ada’s ambition and spirit bring Babbage to draw a new project called “Analytic Engine”, a giant machine made of thousands of cogwheels which could make even more complex calculations with great accuracy. The project lands in Italy in 1842 and Italian engineer Luigi Menabrea writes a scientific article about Babbage’s machine and its functionalities. With a great deal of interest rising in the scientific community, Babbage asks Ada to translate Menabrea’s article into French with the total freedom of adding her personal notes. Ada’s article is three times longer than the original one and her notes show an insightful point of view, along with a deep comprehension of the machine that nobody else had. The most famous part is the “Note G” where she literally develops an algorithm to calculate Bernoulli’s numbers, something that translated into computing could have worked on a modern computer.
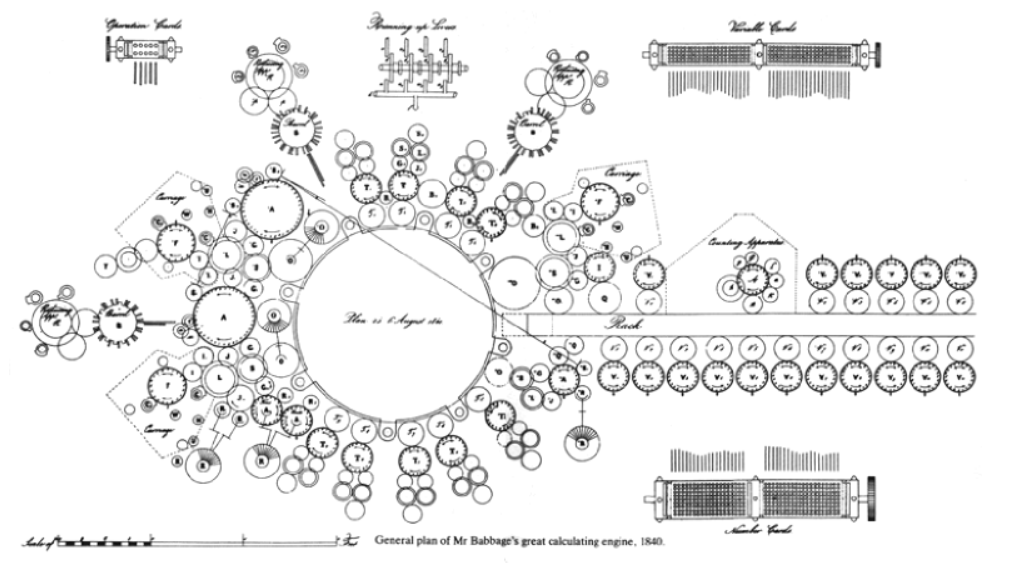
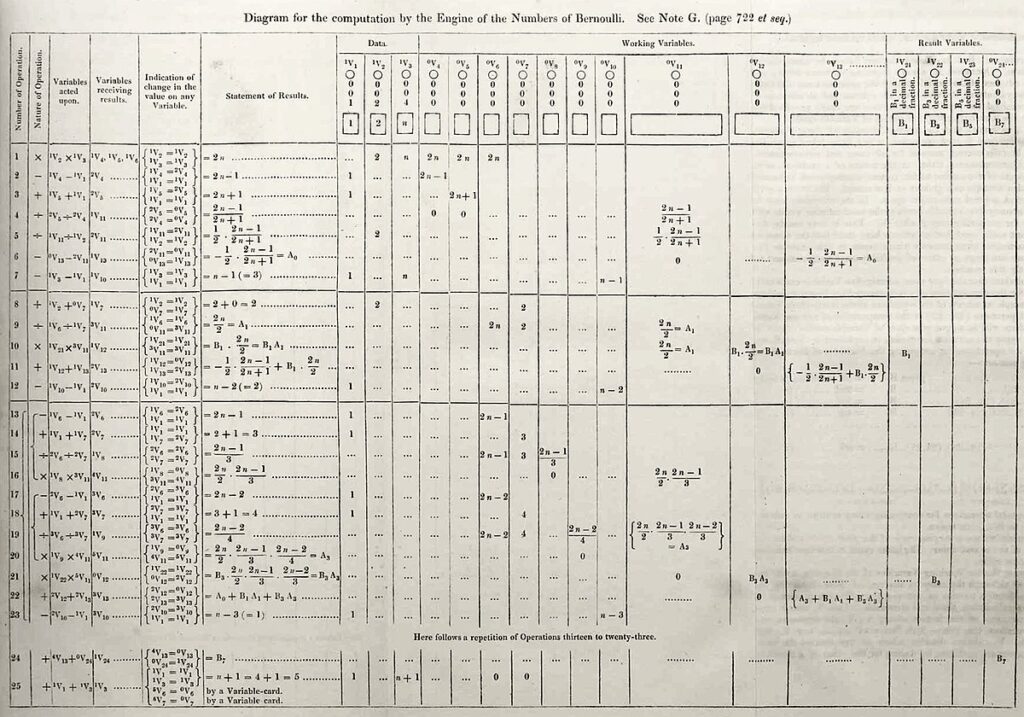
Starting from Babbage’s mechanical calculator (by the way, designed but never actually made), Ada first imagined, then wrote software for an electronic calculator (inclusive of devices, memory and calculation unit) which goes way beyond basic numeric calculation. Her vision has become the starting point for all the computing development in the 1930s and 1940s which brought us to modern technology.
In the 1970s the American Department of Defense developed a coding language called “Ada”, a tribute to the scientist’s foresight. This language is still in use today, especially in contexts where reliability is everything such as aviation or military.
Since 1998, the British Computer Society instituted the “Lovelace Medal” and annually sponsors the “Lovelace Colloquium”, a conference for women undergraduates in digital and tech.

Us too, in the making of Adamantia Group, we wanted to pay tribute to Ada by making it part of our name so that we can always remember where we come from and where we want to go.
A new, a vast, and a powerful language is developed for the future use of analysis, in which to wield its truths so that these may become of more speedy and accurate practical application for the purposes of mankind than the means hitherto in our possession have rendered possible. Thus not only the mental and the material, but the theoretical and the practical in the mathematical world, are brought into more intimate and effective connection with each other.
Ada Lovelace

